Rayleigh Scattering of Isolated Species
( Species == ions, atoms, molecules )
- See Polarization for the low wavenumber (frequency / speed of light ) approximation used for Rayleigh scattering.
Scattering is due to the polarization of species. The polarization can be summed from the behavior of individual resonances and damping factors (related to resonance bandwidth), which I have not yet been able to find. For mostly-isolated atoms in high vacuum, Beers line broadening will not be relevant; the bandwidth 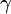
note: perhaps I can get the relevant numbers from HITRAN, but many of the resonances (especially for tightly bound molecules and deep atomic orbitals) will be far into the ultraviolet, where HITRAN may not go. TBD
The scattering from a single resonator at frequency 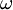
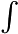 Large
Large 4(
4( 2 −
2 −  02)2 +
02)2 +  2
2 2 =
2 =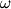
 2
2 
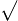
 2 − 4
2 − 4 02
02 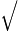

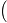
 −
− 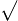
 2 − 4
2 − 4 02
02 − 2
− 2 02
02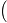 2
2 04 + 2
04 + 2 02
02
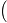
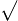
 2 − 4
2 − 4 02 − 2
02 − 2
 +
+  3
3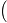
 −
−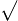
 2 − 4
2 − 4 02
02
 tan−1
tan−1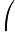


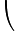
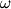
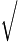 2
2
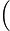
 −
− 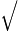
 2 − 4
2 − 4 02
02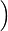 −
−  02
02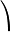


using Wolfram alpha, and slightly reordered for visual symmetry
The actual equation will be a long series of many such terms, one per resonance. And it will really be computed with a C program, which iterates over the resonances, then over the frequency bins and values for the average vacuum solar spectrum. Much information missing, many opportunities for mistakes, and a serious lack of empirical data to compare to.
